Year 8 Maths Chapter 2 - Equations 2
You will want to refer to Year 7 Maths Chapter 9 for basic equations skills. This section is more advanced, and omits algebraic language, basic operations, distributive law, and other basic concepts. It is also suggested you read about Algebraic techniques. For more advanced stuff, you should refer to Year 9 Maths Chapter 2. This section is more basic and omits simulataneous equations
Linear equations are when the power of the pronumeral is 1 and there is only one solution. Another type of equation is the simple quadratic equation, which can be expressed as such:
x^2 = c
The power of the pronumeral is 2. Depending on the value of c, x can have zero to many solutions.
x^2 = c is a simple quadratic equation. If c > 0, then your solutions are as follows:
- x = √c
- X= -√c
If c = 0 there is one solution, which is x = 0.
However, if c < 0 then there aren’t any solutions because x^2 is always positive.
An inequality is a statement of the form:
- LHS > RHS - greater than -
7 > 2 - LHS ≥ RHS - greater than or equal to -
7 ≥ 7, 7 ≥ 2 - LHS < RHS - less than -
2 < 7 - LHS ≤ RHS - less than or equal to -
2 ≤ 2, 2 ≤ 7
Inequalities can be reversed, for example, x < 3 and 3 > x is equivalent. They can also be represented on a number line, as follows.
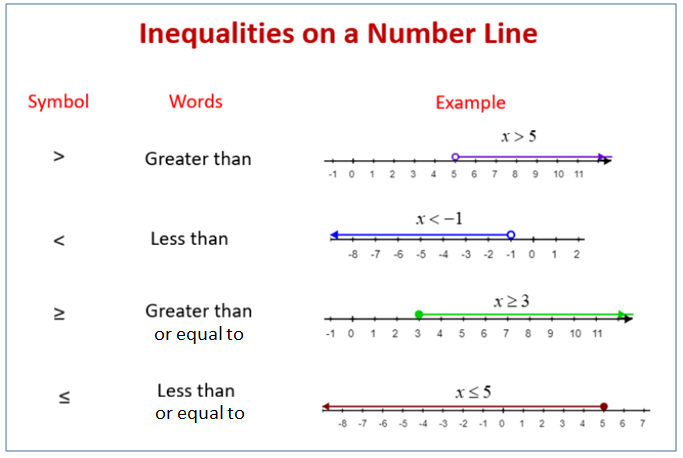
As you can see, the number line is on the x axis, with an arrow above the corresponding numbers. For example, in the first example, you can see that x is larger or equal to than five. As it is inclusive of five, there is a filled circle. On the contrary, with a statement that lacks the ‘or equal to’ statement, it is exclusive, like in the second example, where 6 is excluded, thus the open circle.
Tl;dr: If the statement contains an ‘or equal to’ statement within the inequality, then the circle is open. Otherwise, it is closed.
A range can be represented as a segment on the number line.
Solving inequalities can be performed by finding an equivalent inequality to the given.
- Adding or subtracting an expression from both sides
- Multiplying or dividing both sides by any positive number
- Multiplying or dividing both sides by a negative number AND reversing the inequality symbol
- Swapping LHS and RHS and reversing the inequality symbol
The above represent methods in which an equivalent inequality can be obtained.
Problem solving - Two people painting a wall
You can use equations to answer a question such as the following:
Kingston prepares a rabbit for sacrifice in 8 hours. Oscar does that in 6 hours.
If Kingston and Oscar worked together, how long would it take to sacrifice a rabbit?
Answer:
Kingston prepares ⅛ of a rabbit in 1 hour.
Oscar prepares ⅙ of a rabbit in 1 hour.
∴ together, they prepare ⅛ + ⅙ of a rabbit in 1 hour.
⅛ + ⅙ = 3/24 + 4/24
= 7/24 of a rabbit in 1 hour
Therefore:
One sacrificed rabbit = 24/7
Unfortunately there doesn’t seem to be one easy way to explain why we flip the fraction. You idiot its because you have to divide it by 7 hours to get 1 hour worth of rabbits
Or!
Kingston prepares 3 rabbits in 24 hours
Oscar prepares 4 rabbits in 24 hours
∴ together, they prepare (3 + 4) rabbits in 24 hours.
3 + 4 = 7
= 7 rabbits in 24 hours
Therefore:
One sacrifice rabbit = 24/7